Jay Holben
When the venerable Bill Klages last left the pages of TV Technology, he was discussing some mathematical esoterica regarding inverse square law and I was a bit inspired to pick up, somewhat, from where he left off.
We all know inverse square law as the rule of physics regarding point sources where the intensity of the source will diminish by the square of the distance traveled. For most lighting professionals, this is a rule to determine fall off of a fixture at a specific distance.
YOU DO THE MATH
This works two ways. If you have a fixed relationship between your fixture and your subject, then using inverse square can aid in determining the size of fixture necessary to light your subject to a specific stop. The opposite of this is if you have a fixture that is providing too much intensity, knowing inverse square can help you quickly determine how much light you can reduce by simply increasing the distance to the subject to obtain the intensity you’re looking for.
For the mathematically inclined:
Intensity = 1 divided by the square of the distance.
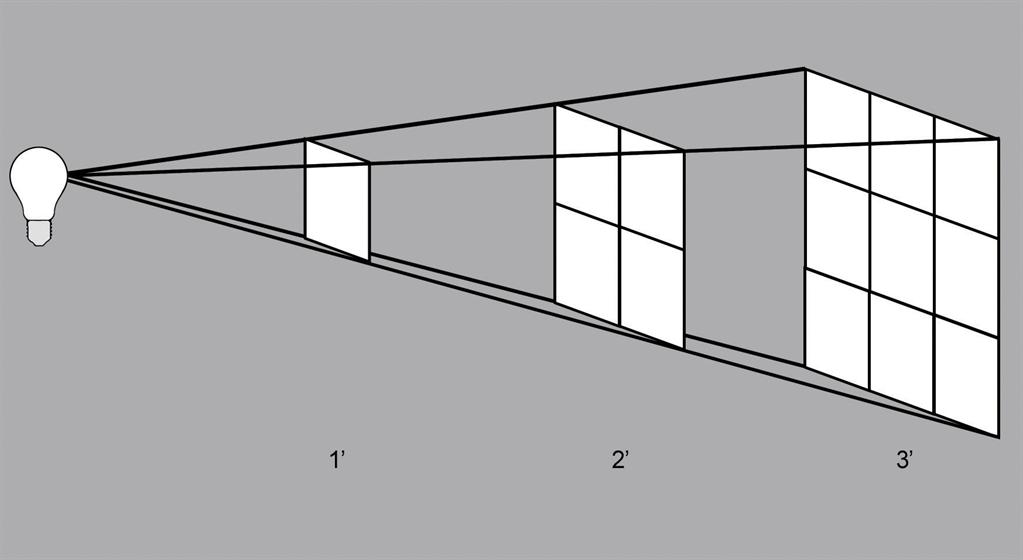
Fig. 1: Light rays emanating from a point source diverge out in all directions equally. The further they travel from the source, the more physical area they cover and the more the overall intensity is diminished.
(Click to Enlarge)
Although the actual formula and hardcore physics only apply to point sources—that is sources without lenses to focus the light, without diffusion, that derive from a single point (such as tungsten lamps or HMI lamps as opposed to fluorescent, a large light source that is not one point, or LED, which are multiple point sources), it can be applied to broader, softer sources as well—although the math will never be as clean. Softer sources will have a greater fall off than just the inverse square.
Looking at some photometrics for lamps, a typical 1K open-face fixture has an intensity of 1,000 footcandles of light at 4 feet. If we increase that distance to 8 feet, our intensity drops to 250 footcandles. We have doubled our distance, but our intensity is now 1/4 (or the inverse square the distance traveled) the output.
If we look at Fig. 1, which is typical to illustrate inverse square, we see that as light rays diverge from a point source, they travel outward in all directions and the further away from the source the more they diverge and cover a larger physical area. So at 1 foot, what might cover a 1-foot square area, at 2 feet will now cover a 4-foot square area. At 3 feet, that same light is now spread out over a 9-foot square area. If we continued to 4 feet, we’d see the light spreading over 16 square feet of area. So from 1 foot to 4 feet, the light has diminished in intensity 1/16 of its initial power because it is spread over that much more area.
ANIMATED SUBJECTS
When you’re dealing with a fixed subject, say a news anchor sitting at a desk or a seated interview subject, the relationship of that subject to the light source doesn’t change. If, however, that interview subject is very animated and leans forward in their chair, their relationship to the light source can change substantially. This is where understanding inverse square can help us alleviate problems before they arise.
If we’re lighting that interviewee with a 4-foot softbox from four feet away and the subject leans forward during the interview, moving one foot closer to the fixture, that subject has changed their relationship to that light by 25 percent—or the reverse of inverse square there’s about a 5 percent increase in intensity on that subject; definitely a noticeable brightness.
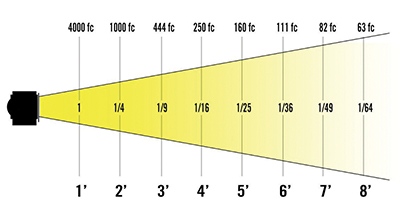
Fig. 2: Illustrating the diminishing intensity of a point source fixture over a given distance. Top numbers represent the theoretical footcandle intensity at that given distance (bottom row of numbers) and the center row of numbers shows the fraction of light – relative to the 1 foot measurement – of light at that point.
(Click to Enlarge)
If, however, you increase the size of the light source to an 8-foot softbox and increase the distance to the subject to 8 feet away, the subjects movement of 1 foot is now only an increase in 1 percent—substantially less.
This is a situation where bigger is better. The 4-foot soft source at four feet has the same quality of light as the 8-foot source eight feet away, but the spatial relationship to the subject is substantially different.
This also works for lighting groups of people— the further away and larger the source is, the more evenly the light will spread among the people. From one to two feet away from a fixture there’s a drop off of 75 percent light intensity, but from that same source, the distances from eight to 12 feet (room for three people to stand) there’s a drop off of less than 1 percent among them.
If we have the ability to increase that source to a 20-foot source 20 feet away, then the intensity difference between our subjects is absolutely negligible and they can move within a substantial area without changing light intensity and exposure.
The practical lesson here is that the larger and further away the source is, the larger an area we can cover with relatively equal illumination from a single source. This is what I like to call the lighting inverse square dance!
Jay Holben is the technical editor of Digital Video and a contributor to Government Video. He is also the author of the book, “A Shot in the Dark: A Creative DIY Guide to Digital Video Lighting on (Almost) No Budget.”
