Lumens for Humans

There will come a time in your lighting career when you will be confronted by a need to determine the number of fixtures you will require to provide at a certain light level.
A typical situation would be one in which you had to light an audience with a load of PAR-64s in a facility where you have had no prior experience. But have no fear—I will address this subject in two consecutive columns.
This first part will cover the basic principles and lay the groundwork for the use of the simple concepts to calculate illumination. So put aside your creative "painting with light" brush and enter the scientist's domain.
Let me lead you through the forest of confusing lighting terms. At this point, there may be a temptation to just resort to simple guesswork and forget science. But be assured that your concentration will be rewarded.
THE CONCEPT OF POWER
In the beginning, we have a light source created by applying electrical power to a lighting device. The concept of power is a simple one—the rate of doing work or, if you will, work per unit time—or, if it is easier to comprehend, energy transmitted per unit time. In electrical terms it is expressed in the familiar unit of watts.
In the case of visible lighting power, lumens are the units used to measure the flow of lighting energy. If we desire the relationship of "power in" to "power out" for an indication of the efficiency of the conversion of electrical power to lighting power, we can describe this by expressing the lumens per watt. The lumen is traditionally defined as the measure of "luminous flux." Forget this 19th-century concept and think of it as "lighting power." More lumens, more light.
An important qualification enters here. When we consider these lighting concepts or photometrics, remember that we are dealing with the vision only, the response of the "typical" eye and its response to the visible spectrum.
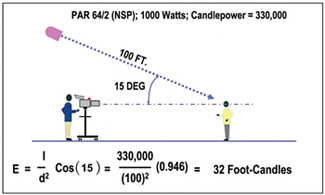
Fig. 1: This diagram illustrates use of candlepower to calculate foot-candles. For the basic simple calculations, the light source must be a "point" source. In other words, it has no dimension, but emits a certain amount of lighting power—lumens. In practical terms, what happens is that a source can be assumed to be a "point" source if the effect of the lighting source is only considered at the distance where the source approaches a point, this generally being at least five times or greater than the apparent size of the source.
I would be disappointed if you are not a little annoyed at this point. Pray tell, why this restriction? The answer is simple. If this were not true, the calculations would become much too complicated to be solved by simple arithmetic formulas.
Because the distribution of the lumens from the source is generally not equal in all directions, we define a second term: "luminous intensity." This is the amount of light leaving our point source in a given direction. It is measured in candelas, or, for the purist, as lumens per steradian. (Respectfully, we ask the purist to be quiet.)
In general practical usage, this is always referred to as "candlepower." The concept is a simple one: the source produces a certain amount of lumens. Depending on the configuration of the lighting unit, the distribution of the lumens will be shaped to meet some application. For example, a spotlight will attempt to concentrate all the lumens in its beam.
Lumens cannot be measured directly, but are important when we are interested in the efficiency of a source alone or, more importantly, when incorporated in a lighting instrument. To describe the distribution of lumens emanating from a fixture, the manufacturer (particularly in the architectural field) will provide the candlepower at many positions about a virtual sphere with the fixture located at the center. This data can be in graphical polar form around horizontal and vertical axes or as a standardized computer text file (.ies) used in many computer-lighting calculation programs.
The number and angles about each axis will vary depending upon the symmetry of distribution. It is not important to this discussion; for our simple calculations, we will only need the maximum candlepower.
A TERM BY ANY OTHER NAME
The third term we are going to define is "illuminance." We are very familiar with this even though I know of no lighting designer who refers to it by its proper name. Usually a designer will refer to the amount of incident light in "foot-candles" or "lux" rather than "illuminance." And, with an apology to the Illuminating Engineering Society, who cares about such details?
The units depend upon what measurement system you are using. Foot-candles are measured in lumens per square foot and lux is measured in lumens per square meter. Of the three units defined so far, it is the only one that can be directly measured. It is what our light meters (using a flat disk) measure when we point it at a source.
One point to be noted at this moment is that if we know (by some divine means) that 100 lumens fall on an area of 2 square feet, the average amount of incident light would be 50 foot-candles (100/2).
If you are wondering when this relationship can be used, I will describe a specific situation in Part 2 of this column.
All of this is leading up to the use of the "square law" formula:

Formula (1) gives us that ability to calculate foot-candles (or lux) at any distance of our point source from the measuring plane. The measuring plane is at right angles to the direction of the candlepower.
What we are really interested in is what our incident light does after it strikes our surface. Again, another assumption is made to simplify our calculation. The subject surface is assumed to be perfectly matte and reflects light in all directions equally, so equal that no matter from where the surface is viewed it appears to be equally bright (a "lambert surface").
It can easily be shown that if we tilt our matte plane away from being perpendicular to the candlepower direction, the incident light must be multiplied by the cosine of the tilt angle (2). It will still appear as equal brightness, but of lesser value, as in tilting we have made the plane bigger. Note that the cosine of 90 degrees is 1.0 (plane perpendicular) and goes to 0.0 as plane becomes in line with candlepower direction.
The calculation of using candlepower to determine foot-candles is illustrated in Fig. 1. The lighted surface is assumed to be measured by a light meter that is pointed towards the camera. For this reason, the cosine of the angle must be applied to account for the direction of the light from the source.
One last thing: when illuminated, our matte surface will return light to the viewer. It is this light that our camera sees and exposes. It will return a portion of the light.
The ratio of reflected light to delivered light is expressed as a fraction or percentage and is termed "reflectance." If we deliver 50 foot-candles (50 lumens per square-foot) and our surface is gray, its reflectance might be 0.20 or 20 percent. The amount of light emitted from the surface would be 10 lumens per square foot. The term for this returned light is "luminous exitance," a term no one uses! Everyone uses the term "brightness" for this quantity and common usage would describe it as 10 foot-lamberts (supposedly a discontinued term) in our example. But it is in common usage; my Spot meter reads "foot-lamberts." Projection experts use foot-lamberts when referring to screen brightness. If you do use the term "brightness" (like everyone does), there is the possibility of being drummed out of the Illuminating Engineering Society (IES). If you are not a member, this threat is meaningless.
We will continue this discussion in Part 2, which will outline practical applications of this information.
For a simple and clear explanation with the mathematical proofs, I suggest the IES Publication "Lighting Metrics TM-9" available from their Web site.
Bill Klages would like to extend an invitation to all the lighting people out there to give him your thoughts atbill-klages@roadrunner.com.
Get the TV Tech Newsletter
The professional video industry's #1 source for news, trends and product and tech information. Sign up below.
